ATILLA CSÖRGŐ – Platonic Geometry

There are only five regular solids in the universe: tetrahedron, cube, octahedron, dodecahedron and icosahedron. The first known description of these solids is found in the writings of Plato, hence the name “Platonic solids”. They all have a “neat” structure: they can all be inscribed within a sphere, and in each solid, all planes, edges and vertices are of the same size. This unique regularity may have inspired Plato to attribute a cosmological significance to these solids: he associated four of the solids with the four elements, while, in his system, the fifth became the foundation of the world.

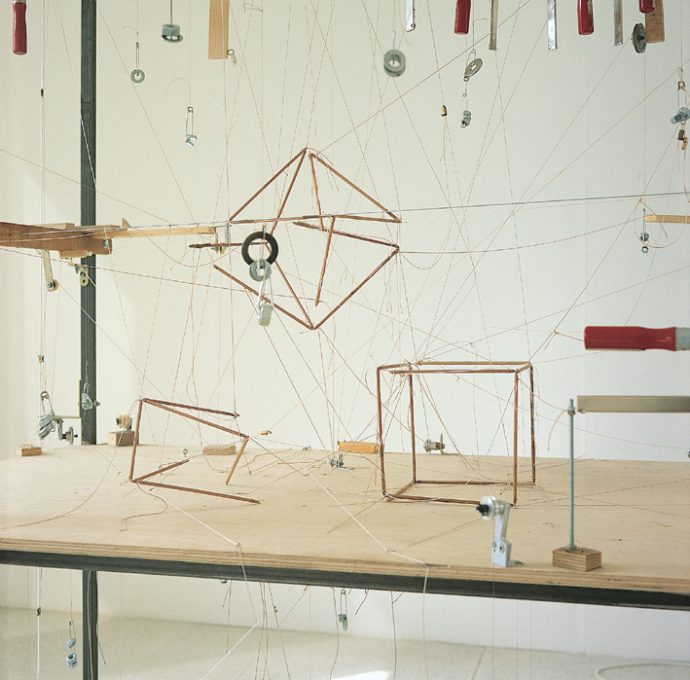
Between 1996 and 2000 I created kinetic construc-tions based on certain geometric correspondences between “Platonic solids”. My starting point was that if the sum of the edges of two solids is equal to the number of edges in a third, then the original solids—put together in the correct way, of course—can be transformed into a different regular shape, regardless of the fact that their other characteristics, such as the number of surfaces or the shape of the geometric planes that provide the sides, differ from one another. Several such transformations can be mapped by counting edges. The most obvious is provided by dual pairs (cube – octahedron and dodecahedron – icosahedron), since, due to the equal number of their edges, their transformability is immediately evident. Among the less obvious examples, we can gain a more complex polyhedron by adding solids with a smaller number of edges, e.g. the combination of a cube and an octahedron gives a dodecahedron, since 6 + 12 + 12 = 30.

I constructed a total of five mobiles that perform the following transformations:

1 tetrahedron + 1 tetrahedron = 1 cube
1 tetrahedron + 1 tetrahedron = 1 octahedron
1 dodecahedron = 1 icosahedron
1 tetrahedron + 1 cube + 1 octahedron = 1 dodecahedron
1 tetrahedron + 1 cube + 1 octahedron = 1 icosahedron

The mobiles were constructed to provide a visual illustration of the “equations”, using simple mechanical solutions such as an electromotor, strings and weights. Initially I had no definite idea about the appearance of these structures; I simply focused on solving the “equation”, that is, on the successful, tangle-free, periodically repeating transformation. In the course of construction, the initial phase of stabilising the frameworks was followed by a longer, improvisational and experimental process. As the elements required permanent restructuring I used readily available materials that were already at hand. This is why I chose to use nuts as weights, and it also explains why there are so many clamps—the result of permanent experimentation with the direction in which the strings were pulling. This temporary, tentative character has been given permanence in the final structures.

The “Platonic solids” represent timeless geome-trical relationships. What connects them here is the temporariness of transformation. The duration of the restructuring between the two end stages is significantly longer than the moment in which the solids appear as distinct forms. This intermediate stage appears relatively unstructured, in that it displays a character which is difficult to define. If the motion were to be frozen in this intermediate stage, there would be little to suggest that the jumble of edges had any connection with a regular solid. The decreasing/increasing confusion of the intermediate forms does not satisfy the topology of regularity; their irregularity is, however, the result of a precisely calculated motion. Extracted from the continuity of motion, they might be regarded as half solids, or the embryonic forms of solids. If one looks instead at the continuity of motion, the whole of the metamorphosis may be regarded as a multi-directional, pulsating body, with two timeless forms at its end stages

Attila Csörgő